Acoustic Feedback (also referred to as the Larsen effect) has been roaming around sound reinforcement systems for a very long time, and everyone seems to have their own way to tame the feedback lion. Digital signal processing opened up the microphone to some creative solutions, each with its own unique compromises. This article takes a closer look into that annoying phenomenon called acoustic feedback and some of the DSP based tools available for your toolbox.
Every typical sound reinforcement system has two responses, one when the microphone is isolated from the loudspeaker (open-loop) and a different response when the microphone is acoustically coupled with the loudspeaker (closed-loop). The measured response of the output of a system relative to its input is called its transfer function. If the measured open-loop response of a system has constant magnitude across the frequency range of interest you can model the system using a level control followed by some delay. Looking at the transfer function of a simple level change and delay element can provide insight into the behavior of acoustic feedback in real world situations.
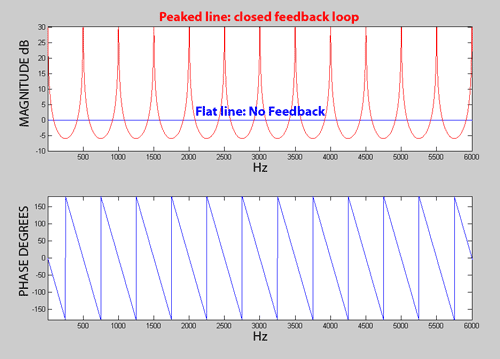
The top half of figure 1 compares two magnitude responses. The flat (blue) line represents the magnitude of an open-loop system (no feedback) with unity gain (0 dB) and 2 ms of delay. The peaked (red) curve is the same system after the feedback loop is closed. The closed-loop has peaks that correspond with zero degree phase locations shown in the lower half of the figure. The closed-loop valleys correspond with the 180 degree phase locations. Feedback is a function of both magnitude and phase. Even though the open-loop gain is the same at all frequencies, only frequencies that are reinforced as they traverse the loop (near zero degrees of phase shift) will runaway as feedback.
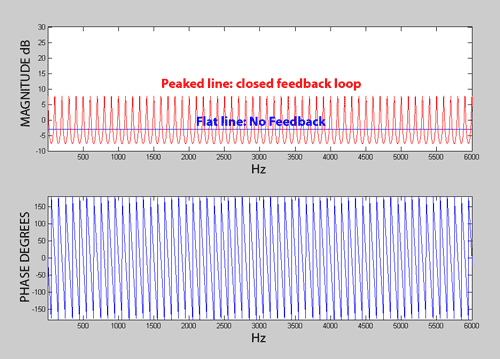
Figure 2 shows the effects of reducing the gain by 3 dB and increasing the delay to 10 ms. Notice that the closed-loop gain reduces significantly (more than the 3 dB of open-loop attenuation that was applied) and that the potential feedback frequencies (areas of 0 degrees phase shift) get much closer together. The zero degree phase locations repeat every 360 degrees of phase change. For a linear phase transfer function you can calculate the frequency spacing of potential feedback locations as a function of delay time. The equation for calculating the delay time is:
Delay Time (sec) = -ΔPhase / (ΔFrequency x 360)
When ΔPhase = 360 degrees (the phase difference between two 0 degree phase locations), this leaves:
ΔFrequency = 1 / Delay Time (sec)
when ΔPhase is 360 degrees
This means that the potential feedback frequency spacing = 1 / delay time (in seconds). The following shows the potential feedback frequency spacing for various delays.
This implies that adding delay makes the potential for feedback worse (i.e. there are more potential feedback frequencies because they are closer together).
Practical experience will tell you otherwise. This is because delay also affects the rate at which feedback grows and decays. If you have 10 ms of delay between the microphone and loudspeaker and +0.5 dB of transfer function gain at a potential feedback frequency, then feedback will grow at a rate of 0.5 dB / 10 ms or +50 dB / second. If you increase the delay to 100 ms then the growth rate slows to +5 dB / second.
Here is another observation regarding gain and its relationship to feedback: For a fixed delay you can calculate the growth rate of a feedback component if you know how far above unity gain the open-loop system is at a particular feedback frequency. This means that if you are at a venue and can hear feedback growing (and can estimate its growth rate) you can calculate roughly how far above unity gain the system is (this also means your kids probably call you a nerd).
As an example if you estimate that feedback is growing at a rate of 6 dB / second and you know that the distance from the loudspeaker to microphone is 15 feet then you know that the gain is roughly only (6 x 0.015) or 0.09 dB above unity gain. So... you only need to pull back the gain by that amount to bring things back into stability.
Of course the rate of change also applies to feedback as it decays. If you pull the gain back by 0.09 dB the feedback will stop growing. If you pull back the gain by 0.2 dB then the feedback frequency will decay at close to the same rate that it was growing. If you reduce the gain by 3 dB (below the stability point of unity) it will decay at a rate of 200 dB / second.
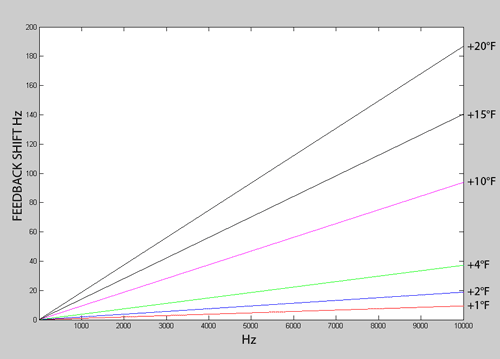
Note also that anything that changes phase will affect the feedback frequency locations. This includes temperature changes as well as any filtering and delay changes. If you analyze how temperature changes affect the speed of sound and look at the corresponding effective delay change that a temperature shift yields, you end up with an interesting graph. Figure 3 shows the shift of a feedback frequency based solely on how temperature affects the speed of sound. The interesting points are that feedback frequency shifts are larger at higher frequencies and the potential for feedback frequency shifts could be significant (depending on your method of control), but more on this later.
To summarize:
Understanding feedback is one thing, taming it is quite another. There are three main methods used by equipment manufacturers for controlling feedback. The Adaptive Filter Model method (similar to a method used in acoustic echo cancellation), the Frequency Shifting method and the Auto-Notching method. Most of this discussion is on auto-notching as it is the most commonly used method.
This method is very similar to algorithms used in acoustic echo cancellation for teleconferencing systems. The idea is to accurately model the loudspeaker to microphone transfer function and then use this model to remove all of the audio sent out the local loudspeaker from the microphone signal.
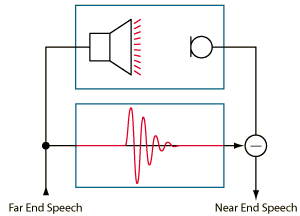
Figure 4 shows a teleconferencing application. The audio sent out the loudspeaker originates from a far-end location, and the removal of this audio from the local near-end microphone keeps the far-end talker from hearing his own voice returned as an echo. The far-end talker's voice is used as a training signal for the modeling. This modeling is an ongoing process since the model needs to match the ever-changing acoustic path.
During this modeling any local speech (double talk) acts as noise which can cause the model to diverge. If the model is no longer accurate then the far end speech is not adequately removed. In fact, the noise added from the inaccurate model can be worse than not attempting to remove the echo at all. Much care is taken to avoid the divergence of the path model during any periods of double talk.
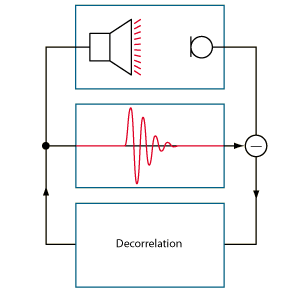
A sound reinforcement application is shown in figure 5. Here there is no far-end speech to feed the model. The local speech is immediately sent out the loudspeaker and is the only training signal available. The fact that the training signal is correlated with the local speech (seen as noise to the training process) provides a significant problem for the adaptive filter based modeling. This is particularly true if it is trying to maintain a model that is accurate over a broad frequency range.
To overcome this problem some form of decorrelation is introduced (such as a frequency shift). This helps the broad band modeling process but adds distortion to the signal. As with the teleconferencing application if the model is not accurate further distortion occurs. The decorrelation, along with any added distortion due to an inaccurate model, makes this method less appealing for some venues. The big advantage to this type of a feedback suppressor is that your added gain before feedback margin is usually greater than 10 dB.
Frequency shifting has been used in public address systems to help control feedback since the 1960's. Feedback gets generated at portions of the transfer function where the gain is greater than 0 dB. The loudspeaker to microphone transfer function, when measured in a room, has peaks and valleys in the magnitude response. In frequency shifting all frequencies of a signal are shifted up or down by some number of hertz. The basic idea behind a frequency shifter is that as feedback gets generated in one area of the response it eventually gets attenuated by another area. The frequency shifter continues to move the generated feedback frequency along the transfer function until it reaches a section that effectively attenuates the feedback. The effectiveness of the shifter depends in part on the system transfer function.
It is worth pointing out that this is not a "musical transformation" as the ratio between the signal's harmonics is not preserved by the frequency shift. A person's voice will begin to sound mechanical as the amount of the shift increases. While "audible distortion" depends on the experience of the listener most agree that the frequency shift needs to be less than 12 Hz.
How much added gain before feedback can be reasonably expected? The short answer is only a couple of dB. Hansler1 reviews some research results that indicate that actual increase in gain achieved depends on the reverberation time as well as the size of the frequency shift. Using frequency shifts in the 6-12 Hz range, a lecture hall with minimal reverberation benefited by slightly less than 2 dB. An echoic chamber with reverberation time of greater than 1 second could benefit by nearly 6 dB by the same frequency shift.
Digital signal processing allows frequency-shifting techniques in a large variety of applications. When used in conjunction with other methods such as the adaptive filter modeling previously mentioned, it can provide an even greater benefit. However, the artifacts due to the frequency shifting are prohibitive in areas where a pure signal is desired. Musicians are more sensitive to frequency shifts, so think twice before placing a shifter in their monitor loudspeaker path.
Automatic notch filters have been used to control feedback2 since at least the 1970's. Digital signal processing allows more flexibility in terms of frequency detection as well as frequency discrimination and the method of deploying notches. Auto-notching is found more frequently among pro-audio users than the other methods because it is easier to manage the distortion.
When considering automatic notching algorithms there are three areas of focus: frequency identification, feedback discrimination and notch deployment.
Frequency identification typically is accomplished by using either a version of the Fourier transform or an adaptive notch filter. Both methods of detection allow the accurate identification of potential feedback frequencies. While the Fourier transform is naturally geared toward frequency detection, the adaptive notch filter can also determine frequency by analyzing the coefficient values of the adaptive filter. However, detection of lower frequencies (less than 100 Hz) are problematic for both algorithms. Fourier analysis requires a longer analysis window to accurately determine lower frequencies and the adaptive notch filter requires greater precision.
There are two main methods used in discriminating feedback from other sounds. The first method focuses on the relative strength of harmonics. The idea is that while music and speech are rich in harmonics feedback is not.
Note that either of the frequency detection methods (Fourier transform or adaptive notch filter) could be used to determine the relative strength of harmonics. It is easier to think in terms of harmonics if you are using a Fourier transform, but just as frequency can be determined by analyzing coefficients so also can analyzing the relationships between sets of coefficients identify harmonics.
There are drawbacks in utilizing harmonics as a means of identifying feedback. First, feedback is propagated through transducers and transducers have non-linearities. This means that feedback (especially when clipped) will have harmonics. Also, feedback does not always occur one frequency at a time. If you remember the discussion on the properties of feedback there is potential for a feedback frequency anywhere the phase of the loudspeaker to microphone transfer function is zero degrees. For a system with 25 ms of delay (roughly 25 ft) this occurs every 40 Hz, and the zero degree frequency locations get closer together as the delay increases. It is not possible to ensure that simultaneous feedback frequencies will never be harmonically related. The potential for feedback with harmonics needs to be balanced against the fact that some non-feedback sounds (tonal instruments such as a flute) have weak harmonics, blurring the area of accurate discrimination.
Another method for discriminating feedback from desirable sound is to analyze feedback through some of its more unique characteristics. This can be done without analyzing harmonic content. For example a temporary notch can be placed on a potential feedback frequency. Feedback is the only signal that will always decay (up stream of the filter) coincident with the placing of the notch. However, because placing a temporary notch is intrusive some other mechanism needs to be used to identify potential feedback frequencies before a temporary notch is placed for verification. One such useful characteristic is that a feedback frequency is relatively constant over the time that its amplitude is growing. This constant frequency combined with a growing magnitude proves very useful as a precursor to the temporary notch.
The final area in auto-notching algorithms is the deployment of the notches. Most auto-notching feedback suppressors allow the user to identify filters as either fixed (static) or floating (dynamic) in nature. This designation refers to the algorithm's ability to recycle the filter if needed. If a feedback frequency is identified the algorithm looks to see if a notch has already been deployed at that frequency. If found the notch will be appropriately deepened. If not found then a new filter is deployed (fixed filters are allocated before floating filters). If all filters are allocated then the oldest floating filter is reset and re-deployed at the new frequency.
Another useful feature is to give the user the option of having the algorithm turn down the broadband gain (with a programmable ramp back time) instead of recycling a floating filter if all filters are used up. Adjusting the broad band gain does not increase the gain margin but it does provide a measure of safety once all of the available filters are gone.
An area in notch deployment that requires careful attention is the depth and width of notches used to control feedback frequencies. To bring a feedback frequency back into stability the system's open-loop transfer function gain simply needs to be below unity at that frequency. A desirable transfer function will have peaks that are reasonably flat through the frequencies of interest. The depth of the notch used to control a feedback frequency should not be greater than the relatively hot area of gain that caused it, plus a little safety margin. This means notches on the order of a couple of dB, not tens of dB. If the auto-notching algorithm is placing notches with a depth of 20 dB or more, something is wrong. One area to look at is the bandwidth of the notches used.
There is a tendency with these algorithms to try and use notches that are as narrow as possible, with the mistaken belief that the cumulative response will be less noticeable. What usually ends up happening is that several narrow notches get placed at a depth of 20 dB or more to lower the overall gain 2 or 3 dB in a larger area. Furthermore, high Q (narrow) notches are less effective at controlling feedback during environmental changes (such as temperature mentioned above) than are low Q (wide), shallow notches. This means if you use low Q, shallow notches you will be less likely to have notches deployed that are not performing any function other then hacking up the hard work you put in on your frequency response. Most auto-notching algorithms allow you to select the default width and maximum depth of the notches used.
How much additional gain before feedback can be achieved from auto-notching? If you had a perfectly flat frequency response then the auto-notching algorithm would not provide any additional gain margin. The best the algorithm can do is pull down the gain in a finite number of locations. If you had a handful of peaks then the auto-notch could provide additional margin based on how much higher the peaks are above the remaining response. Typically the auto-notch provides only a couple of dB of additional gain before feedback.
Despite the lack of large additional gain margin there are still two other significant reasons for having an auto-notch in the system. First, the auto-notch provides a simple tool to aid in the identification of problem spots in the response when the audio system is first installed. Second, it provides a safety net that can remain in place to cope with the ever-changing acoustic path (unwanted additional reflections, gain change etc.).
Acoustic feedback is both a magnitude and phase issue. As such, changes in the system's phase response due to delay, filtering or temperature changes impact potential feedback frequencies. If notch filters are used to control feedback they should be placed after all other changes are made to the system's phase response to ensure their utility. They should also be wide enough to ensure their ongoing usefulness despite changes to the feedback path.
In order to bring a runaway frequency back into stability the magnitude simply needs to be taken below the unity gain mark plus a couple of dB for a safety margin. In addition to a slightly expanded gain margin, the auto-notch tool provides a simple means for ringing out a room as well as leaving a safety net after the original installation is complete.
In addition to auto-notching algorithms, adaptive filter models and frequency shifting algorithms also provide useful ways to suppress feedback and increase a system's gain before feedback margin. An adaptive filter model based feedback suppressor relies on an accurate model of the loudspeaker to microphone acoustic path in order to remove feedback from a receiving microphone. If the model is inaccurate then distortion can occur. A decorrelation process is used to improve the convergence characteristics of the broad band adaptive filter. This decorrelation can also add a limited amount of distortion. However, the adaptive filter model is capable of greater than 10 dB of additional gain before feedback.
The utility of the frequency shifter depends on the system where it is applied. As a general rule the frequency shifter will provide a greater gain margin in a more reverberant space than in a smaller less reverberant space. The frequency shift should be kept to less than 12 Hz to minimize audible distortion.
Acoustic feedback has been roaming around sound systems for some time. The tools just outlined provide a set of unique solutions each with its own compromises. Getting the most out of the tool requires understanding the problem and the proposed solution. With the proper tools in place, perhaps our memories of the howl and screech that characterize the Larsen effect will begin to slowly fade away.
![]() "Understanding Acoustic Feedback & Suppressors" Download this note in PDF.
"Understanding Acoustic Feedback & Suppressors" Download this note in PDF.